What polyhedron can be assembled from this net – Embark on an enlightening journey into the world of polyhedron nets, where we unravel the secrets of transforming two-dimensional patterns into captivating three-dimensional structures. This comprehensive guide delves into the intricacies of identifying polyhedron nets, mastering assembly techniques, exploring their inherent properties, and delving into advanced concepts that govern these fascinating geometric forms.
As we traverse this path of discovery, we will unveil the relationship between the shape of a net and the resulting polyhedron, empowering you to design and create your own unique polyhedron nets. Prepare to be captivated by the elegance and functionality of polyhedrons, as we uncover their applications in architecture, engineering, and beyond.
1. Polyhedron Net Identification
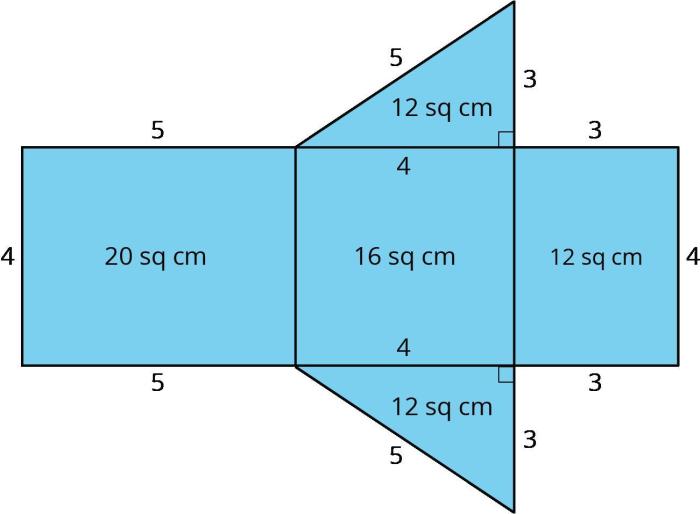
A polyhedron net is a two-dimensional representation of a three-dimensional polyhedron. It consists of a set of polygons connected by edges, which, when folded and assembled, form the polyhedron.
Examples of polyhedron nets include the square net for a cube, the triangle net for a tetrahedron, and the pentagon net for a dodecahedron. The shape of the net determines the faces, edges, and vertices of the resulting polyhedron.
Relationship between Net Shape and Polyhedron
- The number of faces in the polyhedron is equal to the number of polygons in the net.
- The number of edges in the polyhedron is equal to the number of sides of all the polygons in the net.
- The number of vertices in the polyhedron is equal to the number of points where the edges of the polygons in the net intersect.
2. Net Assembly Techniques
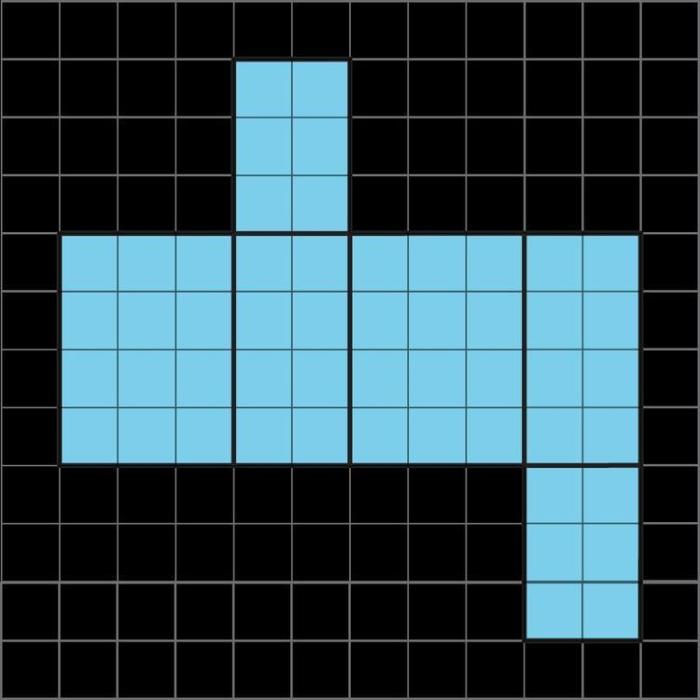
Folding and Alignment, What polyhedron can be assembled from this net
To assemble a polyhedron from a net, precise folding and alignment are crucial. The edges of the polygons should be folded along the creases to form the faces of the polyhedron.
Adhesives and Securing Edges
Once the net is folded, adhesives or other materials can be used to secure the edges of the polygons together. This ensures that the polyhedron retains its shape.
3. Polyhedron Properties and Applications
Properties
- Number of faces: Polyhedrons can have any number of faces, but the most common ones are tetrahedrons (4 faces), cubes (6 faces), octahedrons (8 faces), dodecahedrons (12 faces), and icosahedrons (20 faces).
- Number of edges: The number of edges in a polyhedron is equal to the sum of the number of sides of all its faces.
- Number of vertices: The number of vertices in a polyhedron is equal to the number of points where its edges meet.
Applications
- Architecture: Polyhedrons are used in architectural structures, such as the Great Pyramid of Giza (a square-based pyramid) and the Sydney Opera House (a series of interconnected spheres).
- Engineering: Polyhedrons are used in engineering applications, such as the design of gears and turbines.
- Mathematics: Polyhedrons are studied in mathematics, particularly in geometry, where they are used to investigate properties of three-dimensional space.
4. Net Design and Creation
Geometric Principles
When designing a polyhedron net, it is important to consider the geometric principles that govern the shape and properties of the resulting polyhedron.
Software Tools
Various software tools are available to assist in the design and creation of polyhedron nets. These tools allow users to input the desired shape and properties of the polyhedron and generate the corresponding net.
Factors to Consider
- Number of faces
- Shape of the faces
- Connectivity of the faces
- Desired properties of the resulting polyhedron
5. Advanced Topics
Symmetry
Polyhedron nets can exhibit various types of symmetry, such as rotational symmetry and reflection symmetry. Understanding the symmetry properties of a net can aid in its design and assembly.
Euler’s Formula
Euler’s formula, V – E + F = 2, relates the number of vertices (V), edges (E), and faces (F) in a polyhedron. This formula provides a useful check for the validity of a polyhedron net.
Platonic Solids
Platonic solids are a special class of polyhedrons that are regular and convex. They are named after the Greek philosopher Plato, who studied their properties. There are only five Platonic solids: the tetrahedron, cube, octahedron, dodecahedron, and icosahedron.
Essential FAQs: What Polyhedron Can Be Assembled From This Net
What is a polyhedron net?
A polyhedron net is a two-dimensional pattern that, when folded and assembled, forms a three-dimensional polyhedron. It consists of polygons that represent the faces of the polyhedron, connected by line segments that represent the edges.
How do I assemble a polyhedron from a net?
To assemble a polyhedron from a net, carefully cut out the net and fold along the edges. Align the edges and secure them using glue, tape, or other adhesives to form the desired polyhedron.
What are the properties of a polyhedron?
Polyhedrons are characterized by their number of faces, edges, and vertices. They exhibit various properties, such as symmetry, Euler’s formula, and the relationship between their faces, edges, and vertices.